When Galileo was under house arrest, he could not continue traveling and doing experiments. He was then living with his daughter Virginia who took care of him and recited the daily Hail Mary on his behalf, which was a part of Galileo’s punishment. It was when Galileo could write his another master-piece, On Two New Sciences( 1638), a book that has discussed numbers and infinity. The book is, once again, in dialectical form set between characters of the same name Salviati and Simplicio. Salviati hold the author’s(Galiloe’s) view and the Simplicio is the Aristotalian view holder.

The discussion starts with the divisibility and continuity of matter and space. This leads to a consideration of the number of points on a straight line.Simplicio remarks that under the assumption of infinite divisibility, we should be forced to admit that one infinity may be larger than another, since a long segment contains more points than a short one, though both would be infinite. We now translate quite literally.*

Salviati: These difficulties arise because we with our finite mind discuss the infinite, attributing to the latter properties derived from the finite and limited. This, however, is not justifiable; for the attributes great, small, and equal are not applicable to the infinite, since one cannot speak of greater, smaller,or equal infinities. An example occurs to me which I shall refer to your consideration, Signor Simplicio, since it was you who started the discussion.I take it for granted that you know which numbers are squares and which are not.

Simplicio: Aware of the fact that a square number arises through the multiplication of any number by itself; for example,4 and 9 are square numbers formed from 2 and 3.

Salviati: Excellent. You remember also that just as the products are called squares, the factors, that is, the numbers which are multiplied by themselves, are called sides or roots. The remaining numbers, which are not formed from two equal factors, are called non-squares. If then I state that all numbers, squares and non-squares taken together, are more numerous than the squares taken alone, that is an obviously correct proposition, is it not?

Simplicio: It cannot be denied.

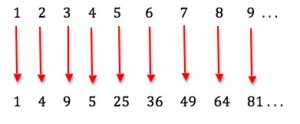
Salviati: If now I ask you that how many square are there, one can nswer with truth, just as many as there are roots ; for every square has a root, every root has a square, no square has more than one root, no root more than one square.

Simplicio: Entirely correct.

Salviati: Again, if I ask how many roots are there, one cannot deny that they are just as numerous as the complete number series, for there is no number which is not the root of some square. Admitting this, it follows that there are just as many squares as there are roots, since they are as numerous as the roots and every number is a root. Yet we said at the outset that all numbers are more numerous than all squares, since the majority of the former are non-squares. Indeed, the more numbers we take, the smaller is the proportion of squares ; for up to 100 there are 10 squares, that is, one tenth are squares ; up to 10000, one hundredth; up to 1000000, only one thousandth. Still up to an infinitely large number, granting we can conceive it, we were compelled to admit that there are just as many squares as numbers.

Simplicio: What is to be our conclusion?

Salviati: I see no escape expect to say: the totality of numbers is infinite, the totality of squares is infinite, the totality of roots is infinite; the multitude of squares is not less than the multitude of numbers, neither is the latter the greater ; and finally, the attributes equal, greater and less are not applicable to infinite, but solely to finite quantities.*

Galileo was the first person in history who introduced the actual infinity and tolerated the consequences in result for the rest of his life. In 1642, after few years of his encounter with Inquisition, he died in 4 January, Arcetri, near Florence. He was the first person to show the fact that whole can be equal to a part of it. He was the one who dared to face the world that had always tried to stop or tortured philosophers from touching infinity. However it was pope John Paul II who ,at the 350th anniversary of Galileo’s death, appologized to Galileo for his treatment by the Inquisition.

* Page 32 in the original edition. An excellent German translation of the entire Discorsi, by von Oettingen, has appeared in Ostwald’s Klassiker,Nos. 11, 24, 25 ; the passage mentioned begins on page 30 of No. 11. The most recent Italian version with commentary will be found in vol. 8 of the Edizione Nazionale of Galileo’s works.